Term Definition secant A line that intersects a circle in two points. Tangent A line that intersects a circle in exactly one point. Average rate of change The average rate of change of a function is the change in y coordinates of a function, divided by the change in x coordinates. Instantaneous rate of change The instantaneous rate of change of a curve at a given point is the slope of the line tangent to the curve at that point. Secant line A secant line is a line that joins two points on a curve. Slope Slope is a measure of the steepness of a line.
A line can have positive, negative, zero , or undefined slope. Together we will walk through three examples and learn how to use the point-slope form to write the equation of tangent lines and normal lines. Thus, equations of the tangents to graphs of all these functions, as well as many others, can be found by the methods of calculus. The geometrical idea of the tangent line as the limit of secant lines serves as the motivation for analytical methods that are used to find tangent lines explicitly. The question of finding the tangent line to a graph, or the tangent line problem, was one of the central questions leading to the development of calculus in the 17th century.
The formulas above fail when the point is a singular point. In this case there may be two or more branches of the curve that pass through the point, each branch having its own tangent line. When the point is the origin, the equations of these lines can be found for algebraic curves by factoring the equation formed by eliminating all but the lowest degree terms from the original equation.
Since any point can be made the origin by a change of variables this gives a method for finding the tangent lines at any singular point. Both Apollonius and de Roberval used geometrical methods to construct tangent lines. One of the first algebraic methods is due to René Descartes, one of the inventors of analytic geometry. It was well known that a tangent line to a circle is always perpendicular to the radius of the circle.
So Descartes' idea was to first find a circle tangent to the given curve. Then the tangent to that circle is the sought after tangent to the curve. An angle refers to the space that is created when two lines intersect or meet. A line that touches the circle at a single point is known as a tangent to a circle.
The point where tangent meets the circle is called point of tangency. The tangent is perpendicular to the radius of the circle, with which it intersects. Tangent can be considered for any curved shapes. Since tangent is a line, hence it also has its equation. In this article, we will discuss the general equation of a tangent in slope form and also will solve an example to understand the concept. To find the equation of a tangent line, sketch the function and the tangent line, then take the first derivative to find the equation for the slope.
Enter the x value of the point you're investigating into the function, and write the equation in point-slope form. Check your answer by confirming the equation on your graph. So far we have found the slopes of two chords that should be close to the slope of the tangent line, but what is the slope of the tangent line exactly? I always like solving advanced problems with basic methods.
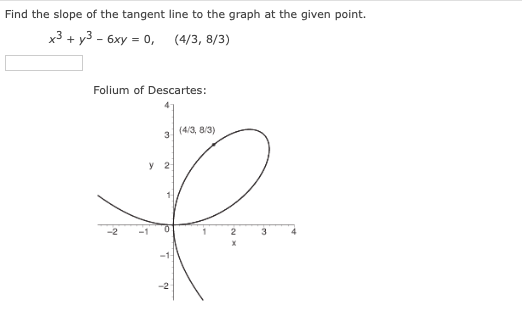
For example, many problems that we usually think of as "algebra problems" can be solved by creative thinking without algebra; and some "calculus problems" can be solved using only algebra or geometry. Using simple tools for a big job requires more thought than using "the right tool", but that's not a bad thing. I want to look at several ways to find tangents to a parabola without using the derivative, the calculus tool that normally handles this task. To determine the x coordinate of the point of intersection of the two tangent lines, we set the tangent line equations equal to one another and solve for x.
As long as mathematicians have been studying curves, they have been interested in the construction of their tangent lines. Apollonius, who lived over two thousand years ago and studied the conic curves extensively, found geometric methods for constructing tangent lines to parabolas, ellipses, and hyperbolas. In fact, Propositions 33 and 34 of Book I of Apollonius' great work, The Conics, give recipes for constructing tangents to these curves. We should note that in the particular case of a circle, there's a simple way to find the derivative.
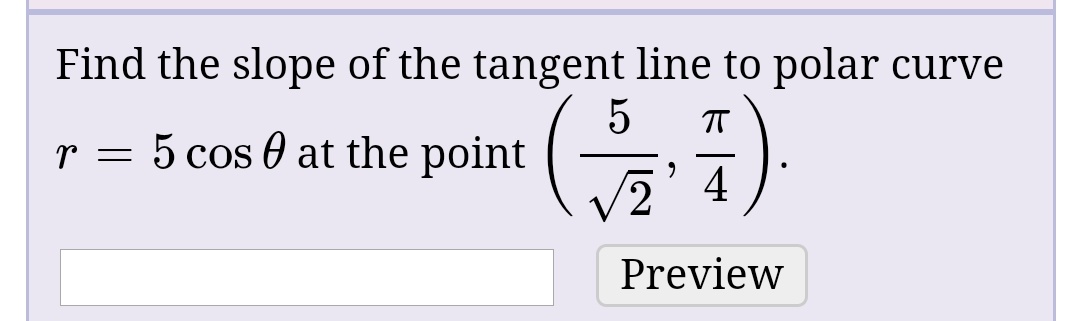
Since the tangent to a circle at a point is perpendicular to the radius drawn to the point of contact, its slope is the negative reciprocal of the slope of the radius. In general, a radius to the point $\ds (x,\sqrt)$ has slope $\ds \sqrt/x$, so the slope of the tangent line is $\ds $, as before. It is NOT always true that a tangent line is perpendicular to a line from the origin—don't use this shortcut in any other circumstance. How tangent lines are a limit of secant lines, and where the derivative and rate of change fit into all this.
The intuitive notion that a tangent line "touches" a curve can be made more explicit by considering the sequence of straight lines passing through two points, A and B, those that lie on the function curve. The tangent at A is the limit when point B approximates or tends to A. You can solve this problem without calculus if you know that a tangent is at right angles to the radius at the point of contact.
Thus the line from the centre of the circle to the point of cantact of the tangent to the circle is perpendicular to the tangent and thus has slope -1. This is not a MATLAB question - it's just analytical geometry formulas and algebra. Just draw it out and make sure you see the right angle between the center, the point off the circle, and the point on the circle. Intuitively, it seems clear that, in a plane, only one line can be tangent to a curve at a point. However, in three-dimensional space, many lines can be tangent to a given point. If these lines lie in the same plane, they determine the tangent plane at that point.
A tangent plane at a regular point contains all of the lines tangent to that point. A more intuitive way to think of a tangent plane is to assume the surface is smooth at that point . Then, a tangent line to the surface at that point in any direction does not have any abrupt changes in slope because the direction changes smoothly. Differentiate the equation of the circle and plug in the values of x,y in the derivative.
Use the slope-point form of the line to find the equation, with the slope you obtained earlier and the coordinates of the point. Unlike a straight line, a curve's slope constantly changes as you move along the graph. To find the equation for the tangent, you'll need to know how to take the derivative of the original equation. As the second value $7+\Delta x$ moves in towards 7, the chord joining $(7,f)$ to $(7+\Delta x,f(7+\Delta x))$ shifts slightly. As indicated in figure 2.1.1, as $\Delta x$ gets smaller and smaller, the chord joining $$ to $(7+\Delta x,f(7+\Delta x))$ gets closer and closer to thetangent line to the circle at the point $$.
You can drag the red dot labeled $f(x+\Delta x)$ to watch this happen; the fixed red line is the tangent line. Likewise, we can even extend this concept to writing equations of normal lines, which are also called perpendicular lines. The only difference will be that we will simply use the negative reciprocal slope of the line tangent. Recall that the roots of this equation represent nothing but the x-coordinates of the points of intersection of the two curves.
If the line touches the circle, then the roots of this quadratic equation must be equal, or coincident. As the circle and parabola lie in the right half-plane, the tangent with the positive slope intersects the curves in the first quadrant. While the tangent with negative slope intersects the curves in the fourth quadrant. Now we have a circle that is tangent to the parabola. We haven't yet found the slope of the tangent line.
To do that without calculus, we can use the fact that any tangent to a circle is perpendicular to the radius. The radius \(\overline\) has slope -2; so the slope of our tangent line is the negative reciprocal, 1/2. Now to find the slope of line K ideally we will require two points on the line, but we know that the radius and the tangent in a circle are always going to be perpendicular to each other. If two lines are perpendicular, the product of the slopes will always be equal to -1.
Apollonius' recipes for constructing tangent lines to conic curves are easy to implement, but his methods were limited in that they could only be applied to a handful of curves. Of course, in the days of antiquity, there weren't that many curves that people were interested in studying, and so there wasn't that much of a need for a general method. Because if we are ever asked to solve problems involving the slope of a tangent line, all we need are the same skills we learned back in algebra for writing equations of lines. Also, do not worry about how I got the exact or approximate slopes. We'll be computing the approximate slopes shortly and we'll be able to compute the exact slope in a few sections.
Find the equation of the tangent line to the circle with center at the origin, at point . Find the equation of the tangent line to the circle with center at (-3,1), at point (-2,5). The tangent plane to a surface at a given point p is defined in an analogous way to the tangent line in the case of curves.
The angle between two curves at a point where they intersect is defined as the angle between their tangent lines at that point. More specifically, two curves are said to be tangent at a point if they have the same tangent at a point, and orthogonal if their tangent lines are orthogonal. These methods led to the development of differential calculus in the 17th century. Further developments included those of John Wallis and Isaac Barrow, leading to the theory of Isaac Newton and Gottfried Leibniz.
A few things have been left unsaid in this lesson – the equation of the tangent in slope form to the circle whose center is not at origin, and the point where the tangent will touch the circle. I calculate the slope of the tangent line according to the fact that the slop of the tangent line is equal to derivation of the circle at that point. Then I have a point off the circle and the slope and I need to find the point on the circle. So I have 2 equations and two unknown variables which are and by solving them I get .
We learned about the equation of a plane in Equations of Lines and Planes in Space; in this section, we see how it can be applied to the problem at hand. I'm trying to create an algorithm to find the tangent on a circle so that I can calculate the angle of reflection for that circle when it collides with an object. I know the x and y values of the centre of the circle and the radius.
I also have the x and y values for the point of impact with the other object. Any help with how to calculate the tangent perhaps using a Java library would be great, or if anyone has any recommendations on how to calculate the angle of reflection another way would be appreciated. The right side of this equation is called the difference quotient. The denominator h is the change in x, and the numerator is the change in y. The beauty of this procedure is that more and more accurate approximations of the slope of the tangent line are obtained by choosing points closer and closer to the point of tangency.
Power transmission – Two circles joined by a pair of mutual tangents can be used to solve the problem of belt length over pulleys. The sum of the length of tangent lines and the arc subtended by them can be used to find the length of the belt. Approximations and differential – The slope of the tangent at a point on a curve defined by the function f can be given by the first derivative f'. The first step is to determine a general formula for the slope of a tangent to the given circle. What is the equation for the slope of a line tangent to the circle? Click the "Submit" button after selecting your answer.
We can use the point-slope formula to determine the equation of the tangent line. Since the circle is centered at , has a radius of 5 and is tangent at (-3, y), using the distance formula we can find the value of y. In this exercise you will use de Roberval's method to construct tangent lines to several curves.
How To Find The Slope Of A Tangent Line Of A Circle Kevin is learning about the basis of calculus and what calculus is actually used for. Unfortunately, Kevin does not understand why calculus is sometimes necessary to find the equation of a line. In Algebra 1, he learned you can find the equation of a line if you are given two points. You find the slope of the line by dividing the up/down difference in the points by the left/right difference, then you use one of the points and the slope to find the y-intercept. Tangent to a circle is the line that touches the circle at only one point.
There can be only one tangent at a point to circle. Point of tangency is the point at which tangent meets the circle. Now, let's prove tangent and radius of the circle are perpendicular to each other at the point of contact. Therefore, let's formally lay out the steps for writing the tangent line equation to a curve, as this particular skill is pivotal for future lessons dealing with linearization and differentials. In reality there probably won't be 15 cm3 more air in the balloon after an hour. The rate at which the volume is changing is generally not constant so we can't make any real determination as to what the volume will be in another hour.
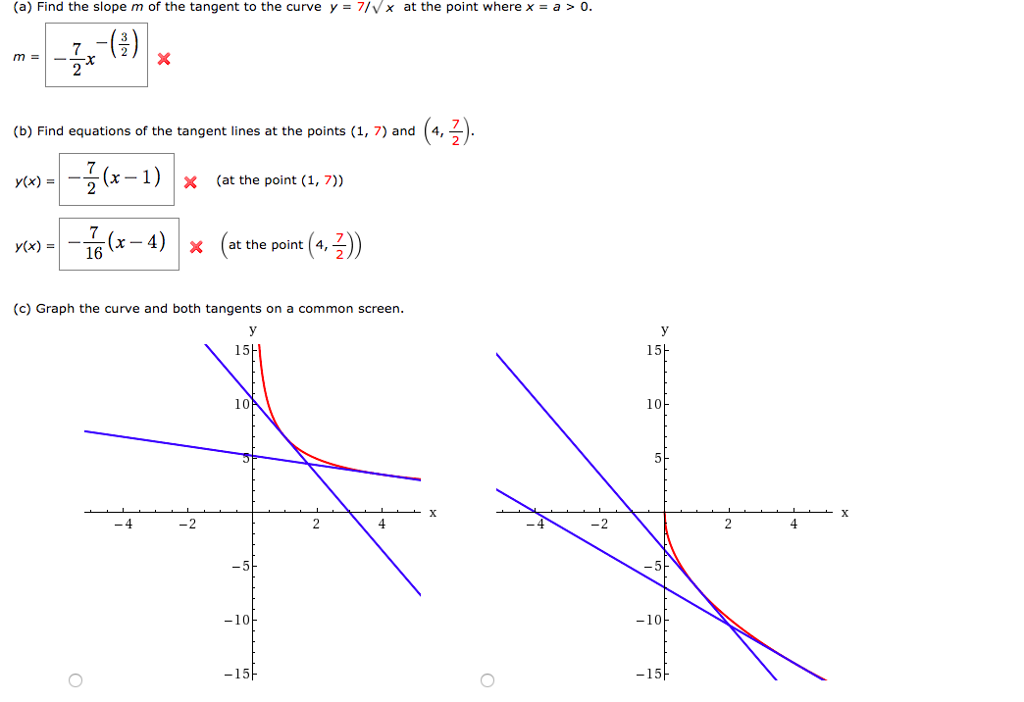
Find the length of the radius of a circle with center at 4,2 and a point on the circle at -8,6. Determine the coordinates of the other endpoint of its diameter. This result is the equation of the tangent line to the given function at the given point. When we have a function that isn't defined explicitly for ??? The slope of the tangent line can be determined by finding the negative reciprocal of this.
In other words, flip the fraction and change the sign. But that's not the final answer because we're trying to find the slope of the tangent line. Since the tangent line is perpendicular to the radius, we can find it by taking the negative reciprocal of the slope of the radius.
Finding the negative reciprocal just means that we flip it over and change the sign. What is the slope of the line that is tangent to a circle at point if the center of the circle is ? Calculating the equation of a tangent plane to a given surface at a given point. The tangent to a circle is perpendicular to the radius, so if you have centre and point of contact, it's very easy to determine the tangent. So here we factored the LHS by using the fact that 2 must be a solution, and therefore \(x-2\) must be a factor, and dividing by that factor using polynomial division.




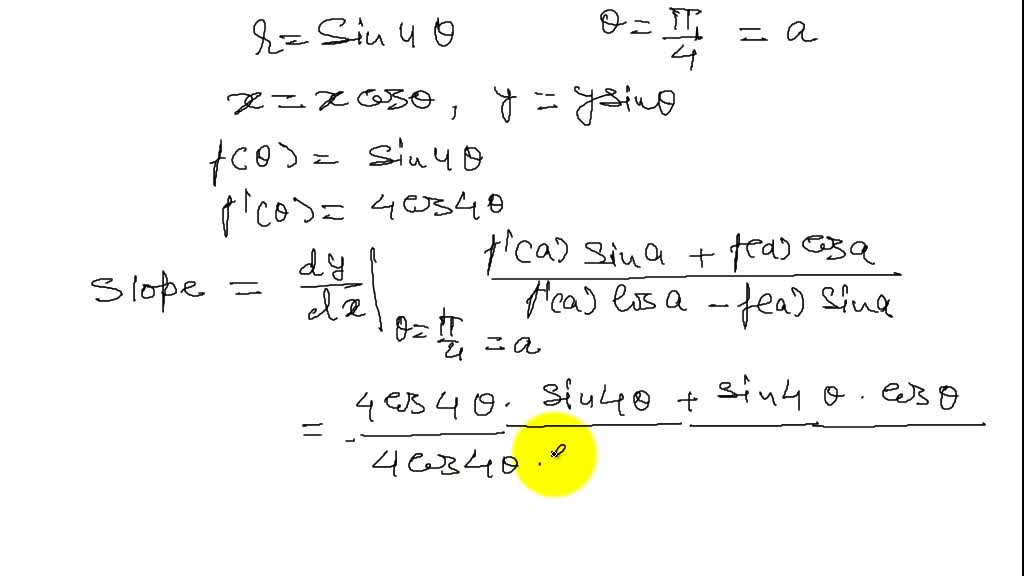



















No comments:
Post a Comment
Note: Only a member of this blog may post a comment.